Modulus Argument Form To Cartesian Form

Wednesday, April 21, 2021

To convert a complex number from polar form to rectangular form you must. The value of z x 2 y 2.

A Complex Number In The Form Is Said To Be In Cartesian Form Complex Numbers What Is There Is No Number Which Squares To Make 1 So There Is No Real

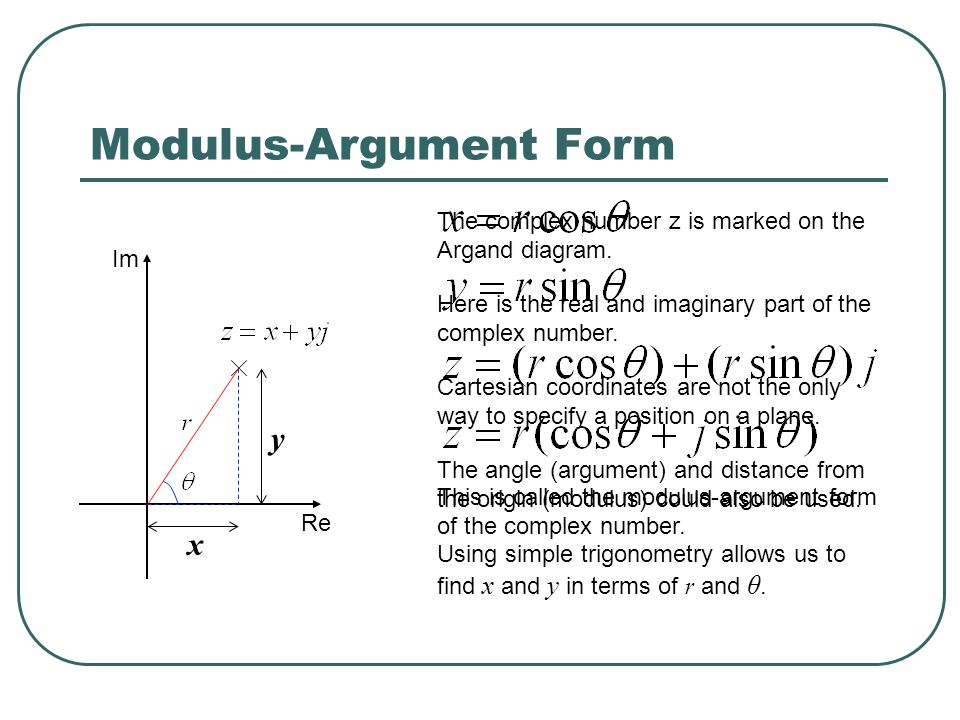
Use the diagram to help you.

Modulus argument form to cartesian form. Construct a triangle in the first quadrant with base 1 height tan θ and therefore hypotenuse sec θ. We must be more careful with the argument. In polar form we write z rθ This means that z is the complex number with modulus r and argument θ.

Step 1 - Find the values of cos30 and sin30 cos30 3 2. To convert into polar form modulus and argument of the given complex number ie. R7 2 -5 2.

Modulus argument form is changed to cartesian form. Z r i cos sin TT where r is the modulus of t he complex number and θ is the argument of the complex number usually expressed in radians. Z a ib Modulus-argument form.

The geometrical representation of complex numbers on a complex plane also called Argand plane is very similar to vector representation in rectangular systems of axes. Multiply and divide complex numbers in modulus argument form. The modulus can be represented as z.

Observe now that we have two ways to specify an arbitrary complex number. Arg Tan-1 yx Tan-1 for arctantan inverse Then put it in the form R cos argisin argshow more. When this happens we are dealing with the polar form.

2 For the complex number express the and coordinates in terms of and. We use Pythagoras theorem in triangle ONP to ﬁnd the modulus of z. The enclosed angle will also be θ since the tan ratio is literally tan θ1.

Convert between the Cartesian form and the modulus-argument form of a complex number. Also the complex values have a similar module that lies on a circle. Finding the Modulus and the Argument of a Complex Number The modulus of a complex number 𝑧 𝑎 𝑏 𝑖 is given by 𝑧 𝑎 𝑏.

Welcome to my HSC 4 Unit maths. The modulus of a complex number in standard form Z a ib is defined by z sqrta2 b2 and its argument theta is defined by tan theta left dfracba right Note Since the above trigonometric equation has. You can use the following rules to multiply complex numbers quickly when they are give in modulus-argument form To prove thse results consider Z 1 and Z 2 in modulus-argument form.

Modulus Root x2y2 R. Complex number times conjugate equals square of modulus proof check 2.

We know the modulus or absolute value of the complex number is given by. The second is by specifying the modulus and argument of z instead of its x and y components ie in the form left rtheta right. Determine the modulus and argument of the complex number Z 2 j3 and express Z i in trigonometric form and ii in polar form Solution Find r and θ r 22 32 49 13 563 2 3 θ arctan i trigonometric form z 13cos563jsin563 ii Polar form z 13563.

This triangle thus gives you the modulus and argument almost straight away without any algebra. Z r θ. Here x0 therefore we will use the formula.

θ c i s θ. Z x iy is the complex number that is in the cartesian form. Is in the 3rd quadrant so we need to add to the acute angle Modulus-argument to Cartesian form Eg.

Knowledge of radians and compound angle formulae is assumed. 1 Convert to form. Note that because arctan xy arctan -x-y you need to be aware of what quadrant your point lies in and potentially adjust by - pi.

Modulus and Argument of a Complex Number - Calculator An online calculator to calculate the modulus and argument of a complex number in standard form. Finding argument of complex number and conversion into polar form. Cos left frac 3pi 8 right frac1sqrt 4 2 sqrt2.

Video - Modulus-Argument Form to Cartesian Form 538 Video - Mod-Arg Form to Cartesian Form in Pure Real or Imaginary 423 Video - Simplifying Complex Numbers using Mod-Arg Form 345. This gives us the more common way to express a complex number in modulus-argument form. Lets try some examples.

To find the argument of a complex number we need to check the condition first such as. This complex number is in modulus-argument form with modulus r 1 r 2 and argument θ 1 θ 2 as required You can derive similar results for dividing two complex numbers given in modulus-argument form. Convert ez to Cartesian form complex numbers 2.

Substitute in those values. We recall the method for finding the modulus and the argument of a complex number in the Cartesian form. About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features 2021.

When the angle θ shown in Figure 3 is measured in a clockwise sense convention dictates that the angle is negative. In this video we see how to convert from cartesian form to modulus argument form and vice versa. θ sec.

Sometimes it is convenient to use the following short-hand notation for a complex number in modulus-argument form. Rzx 2 y 2. Trigonometric or Polar Form.

Instead of starting with the Cartesian form sometimes the modulus r say and argument θ say are given to us. Polar form Z rθ or r θ EXAMPLE 1. Find the values of cosθ and sinθ where θ is the argument.

Let Z be a complex number given in standard form by Z a i The modulus Z of the complex number Z is given by Z sqrt a2 b2 and the argument of the complex number Z is angle theta in standard position. Example 1 - convert z 7cos30 i sin30 to rectangular form. The modulus of the complex number is always positive which is z 0.

One is the standard way x y which is referred to as the Cartesian form of the point. OP 232 2 13 OP 13 Using the symbol for modulus we see that in this example z 13.

Modulus Argument Form Ppt Video Online Download