In Modulo 11 Arithmetic

Tuesday, August 17, 2021

With our list above we nd that this can be done in 2 6 8 96 ways. Modular arithmetic is the branch of arithmetic mathematics related with the mod functionality.

Abstract Algebra 1 Congruence Modulo N Africana Mathematiques Pinterest Algebra And Math

A 2 mod 12 b 4 mod 12 c 19 mod 12 3.

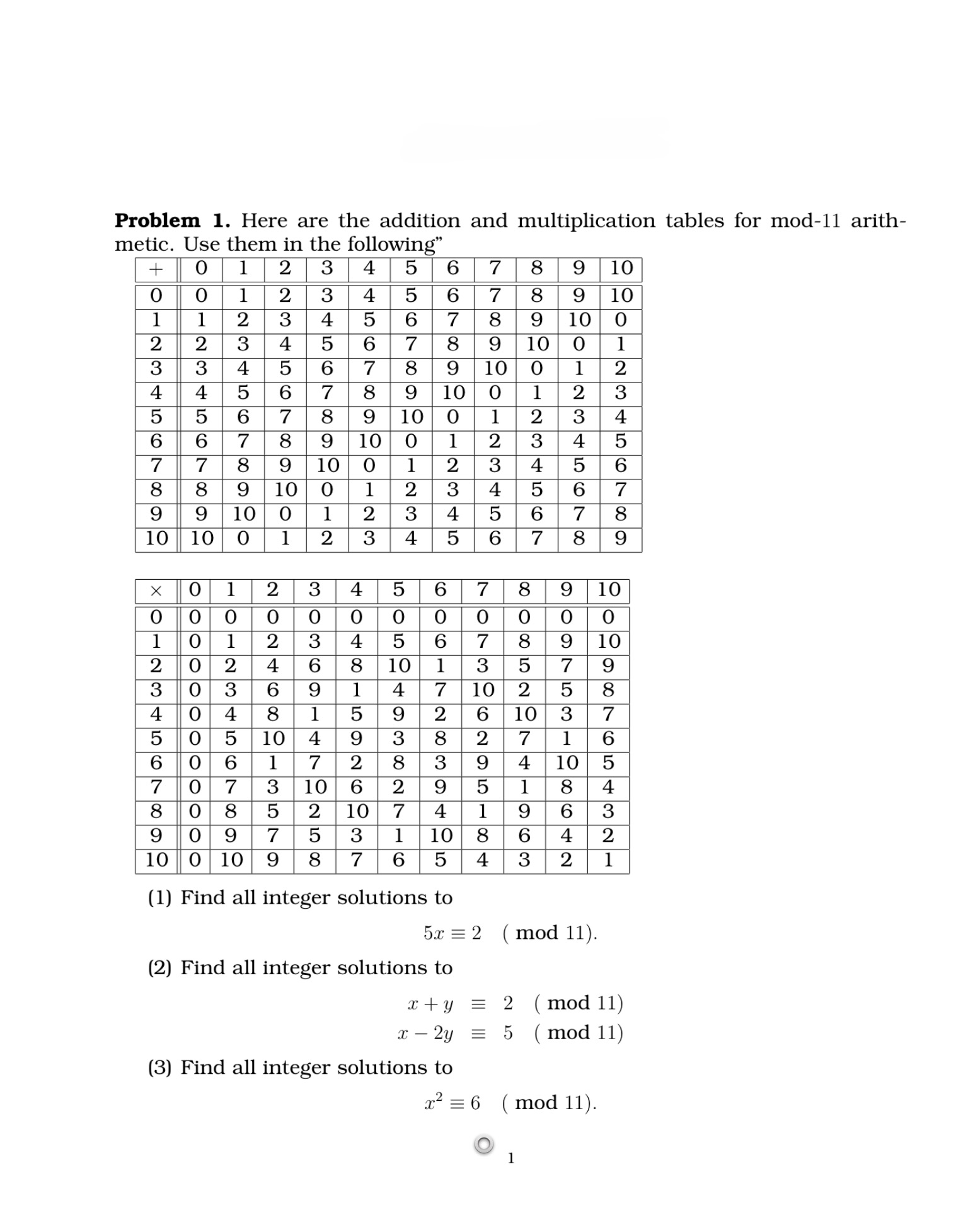
In modulo 11 arithmetic. Many people grow up with the idea that 1 1 2. A classic example of modulo in modular arithmetic is the. This product is divided by the modulus number 11.

For these cases there is an operator called the modulo operator abbreviated as mod. In modular arithmetic we do much the same subject to limitations on division. An Introduction to Modular Math.

We can also divide the clock into 60 equal parts. For example to find 123 321 m o d 11 123 321 pmod11 1 2 3 3 2 1 m o d 1 1 we can take. However in modular arithmetic b may or may not exist.

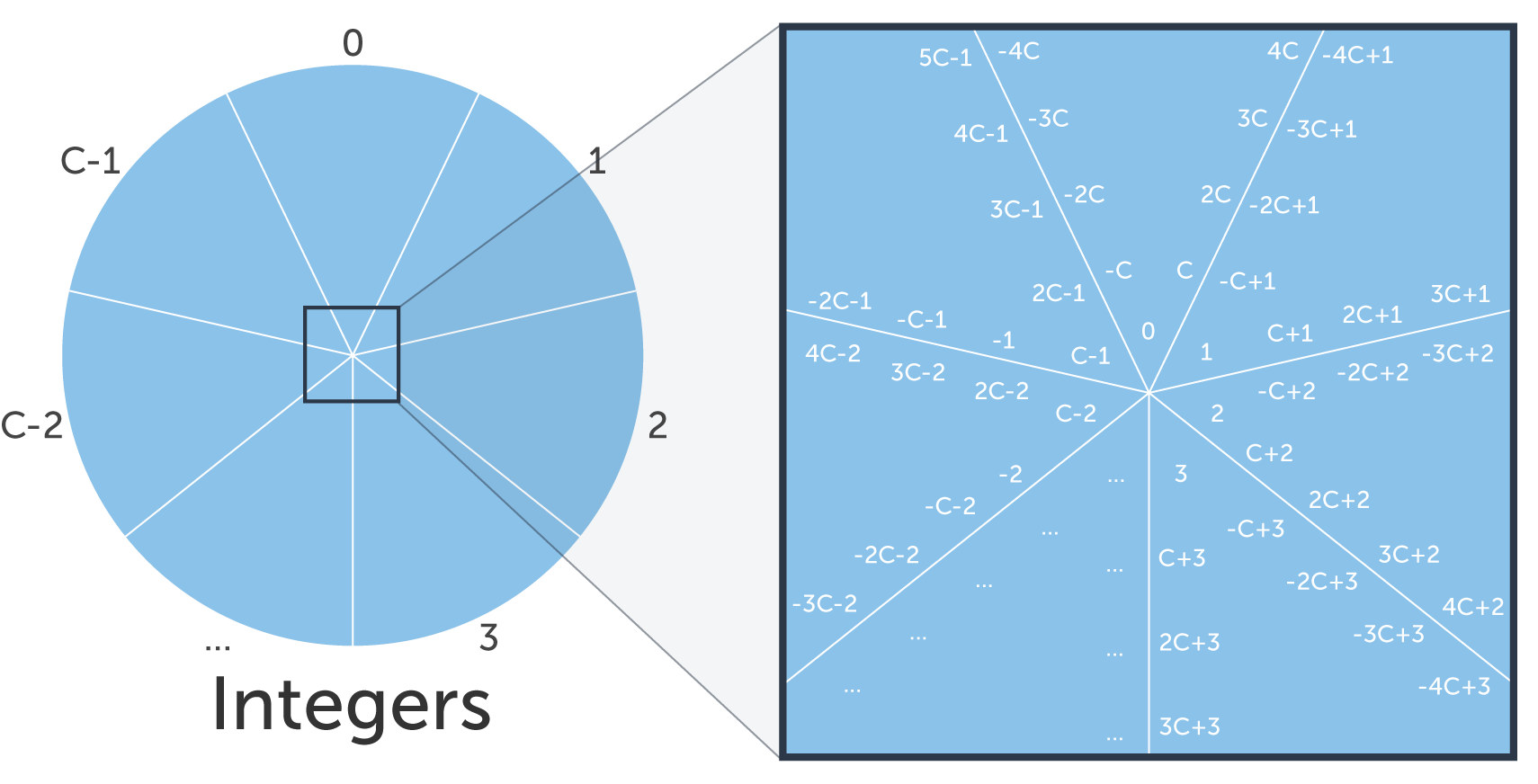
The term modulo comes from a branch of mathematics called modular arithmeticModular arithmetic deals with integer arithmetic on a circular number line that has a fixed set of numbers. In arithmetic the division of two integers produces a quotient and a remainder. They misunderstand the meaning of this equation.

In some sense modular arithmetic is easier than integer arithmetic because there are only finitely many elements so to find a solution to a problem you can always try every possbility. The weights in MOD11 are from 2 through a maximum of 10 beginning with the low order position in the field. Use this to reduce the following numbers in mod 12 arithmetic note that all answers must be between 0 and 11.

To continue the example 140 divided by 11 is 12 with a remainder of 8. Of course that would be all numbers 1ldots 10. So there are 96 residues modulo 1001 that.

Divide sum of products by 11. Modular Multiplication of 11 - Abelian Group. Many complex cryptographic algorithms are actually based on fairly simple modular arithmetic.

On this circle all values are a number from 0 to 11. Most people would never accept the idea that 32 0. First perform the usual arithmetic and then find the remainder.

This allows us to have a simple way of doing modular arithmetic. In modulo-11 arithmetic we use only the integers inthe range ______. So use the Euclidean algorithm to find these numbers.

Each digit in the id is multiplied by its weight. Sometimes we are only interested in what the remainder is when we divide by. When we divide two integers we will have an equation that looks like the following.

Note that while clocks start at 1 and end on 12 modular arithmetic always restarts with zero Conversely when counting down 10 9 8 we follow 0 with 11. Have a unique solution modulo 7 11 13 1001. Well -97 divided by 11 equals -8 remainder -9.

In modulo-11 arithmetic we use only the integers inthe range ______ inclusive. In modular arithmetic the numbers we are dealing with are just integers and the operations used are addition subtraction multiplication and division. In mathematics the result of a modulo operation is the remainder of an arithmetic division.

12 11 132 140 - 132 8 Subtract remainder from 11. The results of the multiplication are added together. If a and m are relatively prime then the.

11 - 8 3. For example 1 11 mod 12 25 10 mod 12. Yes only numbers which are relatively prime to 11 will have an inverse mod 11.

But since this remainder is negative we have to increase our quotient by 1 to say -97 divided by 11 equals -9 remainder 2 as 11-9 2 -97. Each such choice results in exactly one residue modulo 1001. Since math is commonly perceived as having everything right or wrong people will immediately reject the idea of 11 0.

To find the inverse of a number apmod11 must find a number n such that anequiv 1pmod11 or equivalently a pair of numbers such that an11m1. To negative numbers in modular arithmetic. To nd all possible solutions modulo 1001 we need to pick a residue modulo 7 a residue modulo 11 and a residue modulo 13.

All arithmetic operations performed on this number line will wrap around when they reach a certain number called the modulus. Depending on the situation a unit step. Ab1 mod m where a b and m are integers then b is the multiplicative inverse of a.

X divided by y is x multiplied by y-1 if the inverse of y exists otherwise the answer is undefined. 11 minus 8 is 3 so 3 is the modulus 11 check digit for 167703625. A 1to 10 B 1to 11C 0to 10 D none of the above.

Therefore -97 mod 11 equals 2. While we are used to thinking of numbers on an infinite line modular thinking wraps them into a finite number generally represented by a circle. This section provides an Abelian Group using the modular arithmetic multiplication of 11 integer multiplication operation followed by a modular reduction of 11.

For this problem suppose we wanted to evaluate -97 mod 11. We now have a good definition for division. In the last tutorial we demonstrated that the modular arithmetic multiplication of 10 can not be used to define an Abelian Group.

Basically modular arithmetic is related with computation of mod of expressions. Expressions may have digits and computational symbols of addition subtraction multiplication division or any other. 140 11 12 remainder 8 Confirm.

Modular Arithmetic Calculator Math Expressions Arithmetic Modular Arithmetic