By comparing real and imaginary parts of a complex number we get. 1 i c.

Modulus Amplitude Form Complex Numbers Part14 Polar Form Of Complex Numbers Cis Theta Jee Maths Youtube
1 5 0707106807071068i 5 070710678118655 5 070710678118655i 353553391353553391i 353553391 i353553391 3535533935355339i.

Modulus argument form cis. Cis φ cos φ isin φ e iφ. What is modulus argument form. You can derive similar.
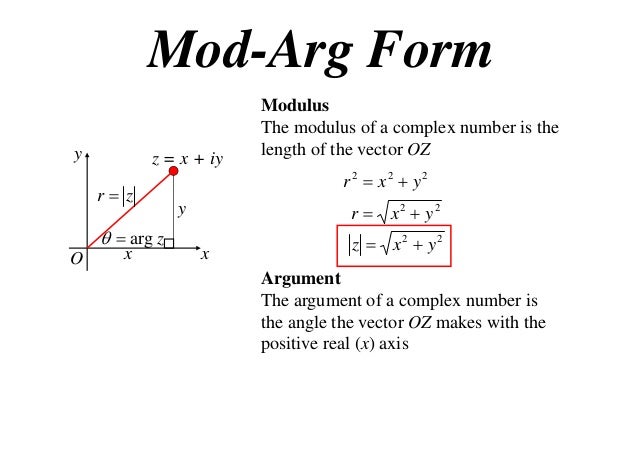
If z x iy is a complex number. B a. This video deals with the modulus-argument or polar form representation of complex numbers and gives examples of how to convert complex numbers from cartesia.
An online calculator to calculate the modulus and argument of a complex number in standard form. There is a special symbol for the modulus of z. Find the modulus and argument of the following complex numbers and write them in trigonometric form.
The reason why we write complex numbers in this form is that there are various properties that allow use to easily perform operations in. The length of the line segment that is OP is called the modulus of the complex number. The notation is less commonly used in mathematics than Eulers formula e ix which offers an even shorter notation for cos x i sin x but cisx is widely used as a name for this function in software libraries.
5 12i d. Convert ez to Cartesian form complex numbers 2. Therefore x iy r cosθ isinθ sometimes written as r cisθ cis cos isin This is known as the modulus argument form.
Z zcosθ i sin θ where θ argz The steps to converting a complex number into polar form. Let Z be a complex number given in standard form by Z a i The modulus Z of the complex number Z is given by Z sqrt a2 b2 and the argument of the complex number Z is angle theta in standard position. The modulus-argument form of a complex number consists of the number which is the distance to the origin and which is the angle the line makes with the positive axis measured clockwise.
I attempt to obtain seven solutions in modulus argument form M1 z textcisleft frac2kpi 7 righttext k 0text 1 ldots 6 A1 ii w has argument frac2pi 7 and 1 w has. Let α tan 1. Then z r cosθ sinθ is called polar form or.
We also have an abbreviation for argument. We first need to find the reference angle which is the acute angle between the terminal side of and the real part axis. To prove thse results consider Z 1 and Z 2 in modulus-argument form.
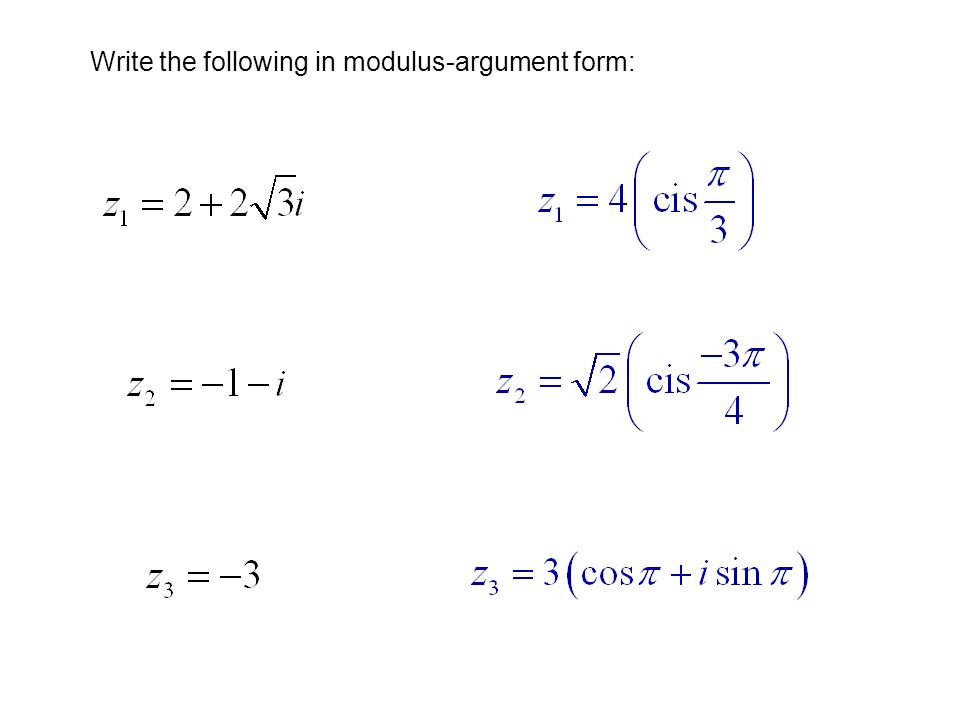
2 cis157079633 i We assume trigonometric angle argument in radians. A textbf a a 4 4 Modulus of the complex number arg 4 0 Purely real numbers lies on the R axis 4 4 c i s 0 Polar form begin align 4 4 text Modulus of the complex number arg 4 0 circ text Purely real numbers lies on the R- axis therefore quad 4 4 mathrm cis. 3 3 i 3 3 i.
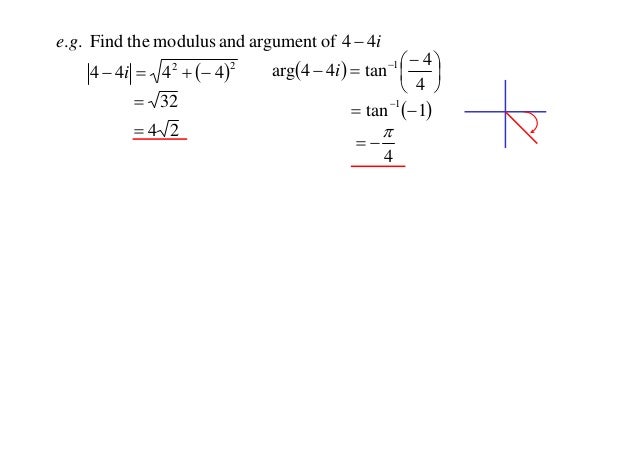
To summarise the modulus of z 43i is 5 and its argument is θ 3697. The value theta is called the argumentof z denoted byoperatornameargz. A 0 b 0.
5 8i b. 5 the result of step No. Hence - 2 i 2 2 cos Π3 i sin Π3 Example 3.
Solution -1 - i 3 r cos θ i sin θ ----1 Finding modulus. Cis45 0707106807071068i We assume trigonometric angle argument in degrees. X r cosθ 1 y r sinθ 2.
Trigonometric form of a complex number. When the complex number lies in the first quadrant calculation of the modulus and argument is straightforward. Cis φ cos φ isin φ e iφ.
Cos left frac 3pi 8 right frac1sqrt 4 2 sqrt2. R -1 2 -3 2 r 1 3 r 4 r 2. Cis is a mathematical notation defined by cis x cos x i sin x where cos is the cosine function i is the imaginary unit and sin is the sine function.
Find the modulus and argument of the complex number-1 - i 3. In polar form the modulus and argument are used to rewrite the complex number in the form. 1 3i 2.
The modulus of is the length of the vector representing the complex number. You can use the following rules to multiply complex numbers quickly when they are give in modulus-argument form. If z1 2 cis 30 and z2 4 cis -60 Find in modulus argument form z.
In this case the modulus is just r b and θ π 2 if b 0 3 π 2 otherwise. FSc Ist yearSecond Year Math Mcqs. Modulus 2 and argument Π3.
Finding argument of complex number and conversion into polar form. The modulus and argument are. This complex number is in modulus-argument form with modulus r 1 r 2 and argument θ 1 θ 2 as required.
Express each of Log On Ad. Complex number times conjugate equals square of modulus proof check 2. The argument is the angle in counterclockwise direction with initial side starting from the positive real part axis.
Suppose that a 0. The angle from the positive axis to the line segment is called the argument of the complex number z. We write argz3697.
X z2 i ii 3 Z1 Z2. The following table shows the value of the argument θ depending on the signs of a and b. The result of step No.
Product Theorem r1 cis 1 r2 cis 2 r1r2 cis 1 2 Quotient Theorem r1 cis 1 r2 cis 2 r1 r2 cis 1 2. The steps to converting a complex number into polar form are. A complex number z in polar formis given asrcos theta isin theta and is oftenabbreviated as roperatornamecis theta where r equals the modulus of the complex number.
The angle can take any real value but the principal argument denoted by Arg is defined as or There are two forms of a complex number. So in this example z 5. Doing this conversion on the calculator requires radian mode argument and the radicals of course give decimal numbers.
Product and Quotient Theorems The advantage of polar form is that multiplication and division are easier to accomplish. Cisthe result of step No.

Complex Powers And Roots De Moivre Applications Ib Maths Hl
Complex Numbers Simplify Answer Simplify Answer Simplify Answer Ppt Download