Hence z ki1ki-1 1 as both numerator and denominaor equals 1k212 Hence z 1. We define the scalar product of a and b as follows.

Misc 15 Find Modulus Of 1 I 1 I 1 I 1 I
The Modulus is the remainder of the euclidean division of one number by another.
If modulus of 1+i/1-i. A z 1 i z 1 i. The modulo operation can be calculated using this equation. Here the critical points are 1 and 2.
Since the real part is 0. 15 which produces the result. This is a differential equation that can be solved with seperation of variables.
Is called the modulo operation. In mathematics modular arithmetic is a system of arithmetic for integers where numbers wrap around when reaching a certain value called the modulus. X 1 2 x 1 -x 2 10.
3 i15 8432 5376i2 3 i. Example 1 Plot the complex number Z -1 i on the complex plane and calculate its modulus and argument. Solution to Example 1 The complex number Z -1 i a i b hence a -1 and b 1 Z is plotted as a vector on a complex plane shown below with a -1 being the real part and b 1 being the imaginary part.
3 i15 3 i16 3 i. SIm eib e iN 2 e i 2 eiN 2 e iN 2 e e i 2 Im eibe iN 1 2 sinN 2 sin 2. The outputs are the modulus Z and the argument in both conventions theta in degrees and radians.
I know that a unitary matrix can be defined as a square complex matrix A such that AAAAI where A is the conjugate transpose of A and I is the identity matrix. Two vectors a and b drawn so that the angle between them is θ. If z x iy Real part x Imaginary part y.
It is true because x 1 x 2 y 1 y 2 are all real and squares of real numbers are 0. If you bought it separate then you should see it on your receipt if you dont have a receipt. The sync 2 has its USB port activated for local storage.
1g dg i dx. Other geometric properties used in design include area for tension radius of gyration for compression and moment of inertia for stiffness. 3 i15 3 i2222 3 i.
For the given complex number z x iy. 5 divided by 7 gives 0 but it remains 5 5 7 5. F z 3 z 3.
The modern approach to modular arithmetic was developed by Carl Friedrich Gauss in his book Disquisitiones Arithmeticae published in 1801. Use of the calculator to Calculate the Modulus and Argument of a Complex Number 1 - Enter the real and imaginary parts of complex number Z and press Calculate Modulus and Argument. G C 3 e i x.
9 given above for sin and cos. Solve for x x 1 x 2 10. You should be able to find a model number on the bottom.
Section modulus is a geometric property for a given cross-section used in the design of beams or flexural members. Square of Real part x 2 Square of Imaginary part y 2. To calculate the value I think it may be easiest to square 3 i four times then divide by 3 i as follows.
2 x 1 x 2 2 y 1 y 2 -. Or -x 1 x 2 10. 1 abs1-i 1-i 1 2 -1 2 14142136 The absolute value of a complex number also called the modulus is a distance between the origin zero and the image of a complex number in the complex plane.
3 i15 8 6i222 3 i. Or -1 10 which is not possible Case 2. The given complex number is 1 7 i 2-i 2 1 7 i 4 i 2-4 i 1 7 i 4-1-4 i 1 7 i 3-4 i As we know that i 2 -1 Now rationalising the denominator we get 1 7 i 3-4 i 3 4 i 3 4 i 3 21 i.
Let z-1 z1 ki where k is any nonzero real. Multiply both sides by - 1 2. Section Modulus Equations and Calculators Common Shapes.
This is referred to as the Polar form of the point. D z 3 4i z 3 4 i. The modulo operation is to be distinguished from the symbol mod which refers to the modulus one is operating from.
3 i15 28 96i22 3 i. 1 e iN 1 e i. Here 9 4 2 and 9 4 1.
Misc 15 Find the modulus of 1 1 1 1 First we solve 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 2 1 21 1 Using a2 टच. Furthermore for a square matrix A the eigenvalue equation is expressed by Avlambda v. To do this we extract a factor exp iN 2 from the numerator and exp i 2 from the denominator of the fraction appearing in Eq.
In computing the modulo operation returns the remainder or signed remainder of a division after one number is divided by another. For x 1-x 1 -x 2 10. Key Point The scalar product of a and b is defined to be ab ab cosθ where a is the modulus or magnitude of a b is the modulus of b and θ is the angle between a and b.
G C 2 e i x. E z 2i z 2 i. Find the square of x and y separately.
So we need to determine what value if any of the constant C 3 makes g x f x. 15 The next step is to try to make use of the formulae Eq. - x 1 x 2 y 1 y 2.
Ln g i x C. Proof of the Triangle Inequality 3. Strength of Materials Beam Deflection and Stress.
Find the real and imaginary parts x and y respectively. If you got it with XT2s then its a sync 1. 1g dg i dx.
C z 33i z 3 3 i. Find the sum of the computed squares. 3 i15 42197248 90660864i 3 i.
For instance 9 divided by 4 equals 2 but it remains 1. Z x 2y 2. G e i x C e C e i x.
Absthe result of step No. If you got the Sync module with Blink Outdoor cameras then you have the Sync 2 for sure. This Module contains guidance for the establishment and maintenance of quality assured pharmacovigilance systems for marketing authorisation holders competent authorities of.
Given two positive numbers a and n a modulo n is the remainder of the Euclidean division of a by n where a is the dividend and n is the divisor. Show that the eigenvalues of a unitary matrix have modulus 1. Let us check for the values less than 1 between 1 and 2 and greater than 2.
The modulus of a complex number is just so the value of the modulus is 1. Plot the following points on a plane and evaluate their polar forms. B z 1 2i z 1 2 i.
A familiar use of modular arithmetic is in the 12-hour clock in which the day is divided into two 12-hour periods. First we solve 1 2𝑖1 3𝑖 Let 𝑧 1 2𝑖1 3𝑖 Rationalizing the same 1 2𝑖1 3𝑖 x 1 3𝑖1 3𝑖 1 2𝑖 1 3𝑖1 3𝑖 1 3𝑖 1 1 3𝑖 2𝑖 1 3𝑖. 0 y 1 x 2 x 1 y 2 2 2 x 1 2 x 2 2 2 y 1 2 y 2 2.

Misc 10 If Z1 2 I Z2 1 I Find Z1 Z2 1 Miscellaneou

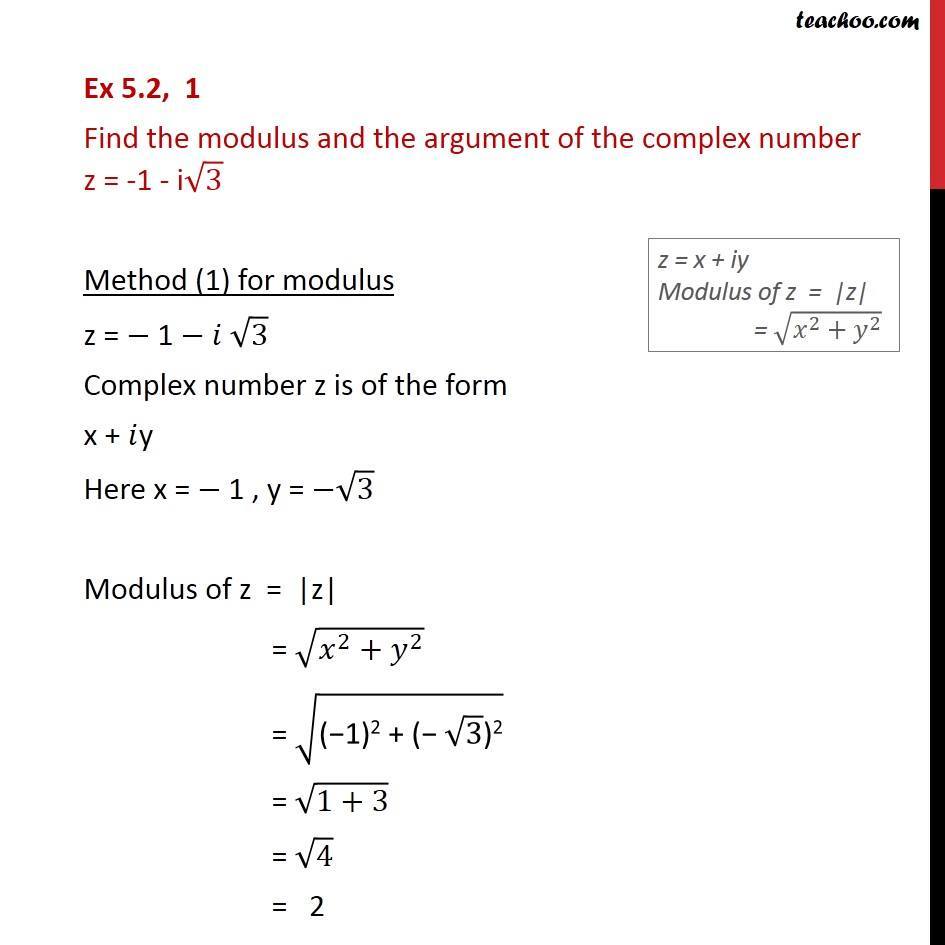
Ex 5 2 1 Find Modulus And Argument Of Z 1 I Root 3
How Do We Find The Modulus Of 1 I From Complex Numbers Quora

Misc 15 Find Modulus Of 1 I 1 I 1 I 1 I

Misc 15 Find Modulus Of 1 I 1 I 1 I 1 I
